Exploring the Fascinating World of Fractals and Recursion
Written on
Chapter 1: Understanding Fractals
Fractals are intricate structures that exhibit self-similarity across various scales. Picture a space where you continuously zoom into a geometric design, revealing smaller versions of the same pattern recursively.
The featured image showcases Romanesco broccoli, a natural representation of fractals with its self-similar patterns. You can see that its smaller components mirror the entire structure, illustrating the concept beautifully.
How Do Fractals Function?
Fractals can become quite complicated, so this discussion aims to simplify the concept while promoting a deeper understanding. We will explore the mathematical ideas of fractions and geometry. If these subjects seem daunting, don’t be alarmed; combining them often makes the concepts clearer and more accessible. Let's dive in!
Section 1.1: The Connection Between Fractions and Geometry
Consider the shaded equilateral triangle depicted below, which covers an area of 1 square unit.
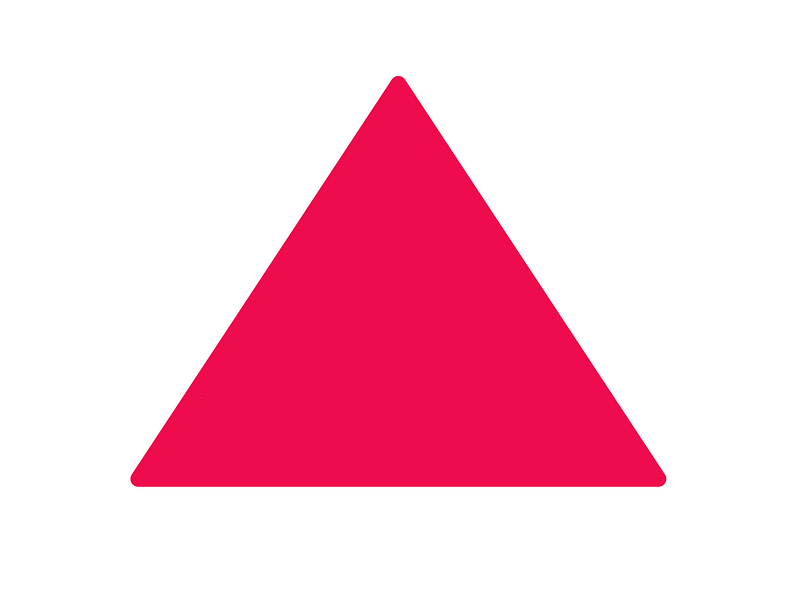
Now, let’s remove a section of this triangle:
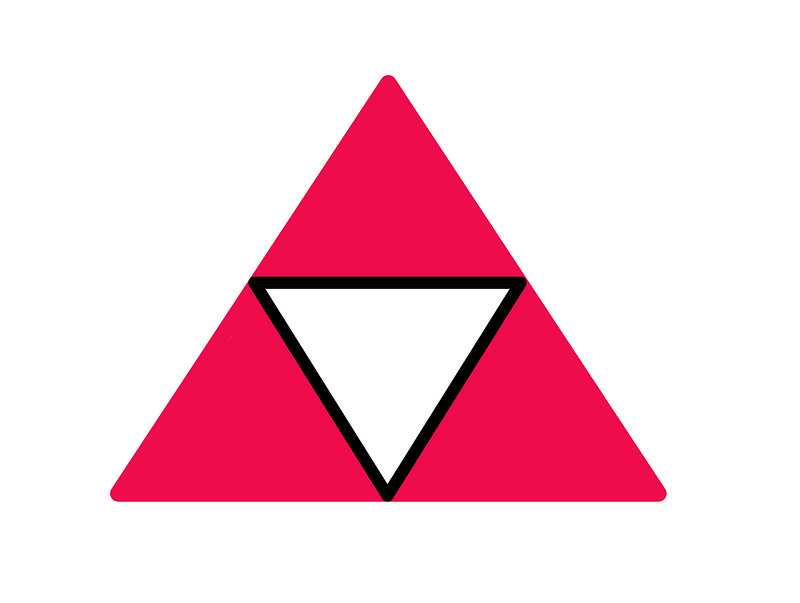
As illustrated, introducing an inverted triangle divides the original triangle into four equal parts. By eliminating the central section, we are left with three-fourths (¾) of the original triangle's area.
Section 1.2: Exploring Fractional Reduction
What happens if we multiply ¾ by ¾? Is the result smaller or larger than ¾? This can be tricky for those not familiar with fractions. However, visualizing it helps.
Multiplying the original area (1 unit²) by ¾ asks: What is three-fourths of this area? When we multiply ¾ by itself, we inquire: What is three-fourths of three-fourths?
This can be visualized in our triangle:
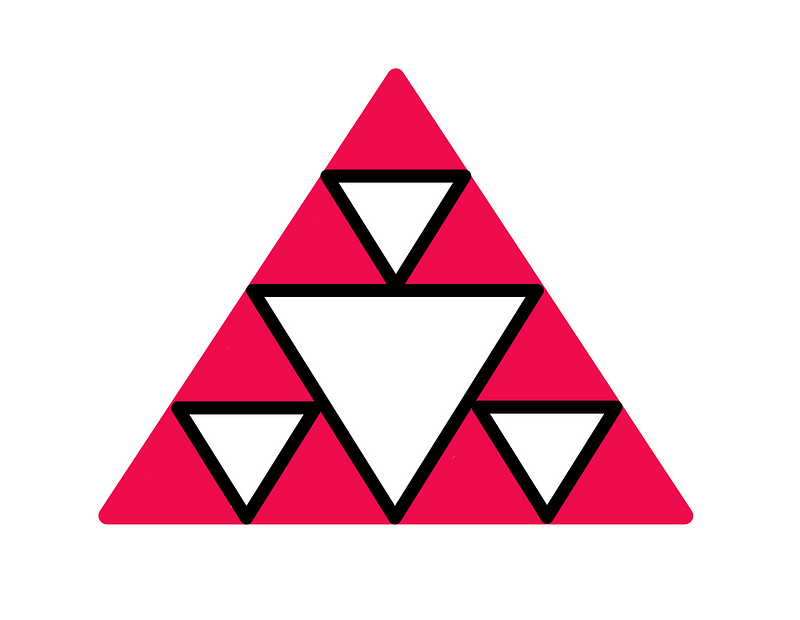
After taking out one-fourth from each of the smaller shaded triangles, we end up with nine smaller triangles. If you count them, you’ll find that the original triangle can be reconstructed using 16 of the smallest triangles, leading to:
(¾)*(¾) = (3²)/(4²) = 9/16.
Every time we multiply by ¾, the resulting figure decreases, illustrating the concept of fractional decay.
Chapter 2: The Sierpiński Triangle
Now that we’ve grasped fractional decay, let's delve into a more advanced idea: the Sierpiński triangle. If we continue multiplying by ¾, our triangle evolves into the following structure:
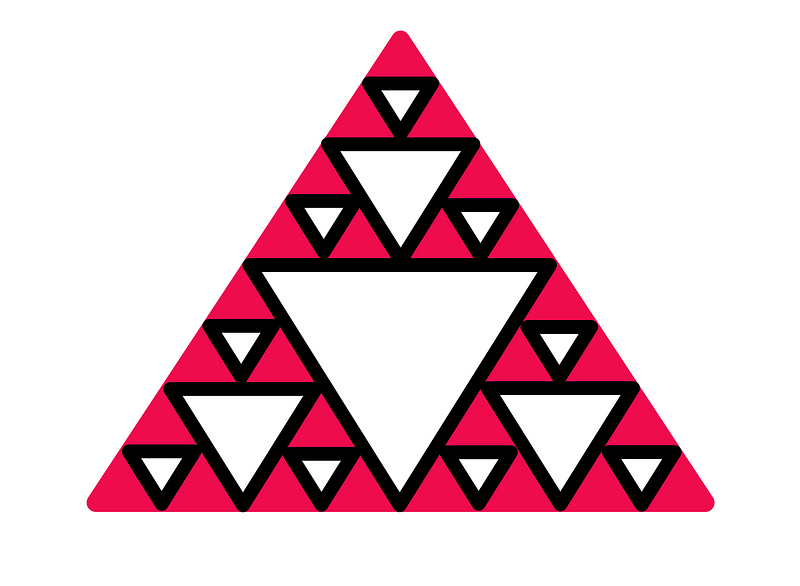
This is known as the Sierpiński triangle, first introduced by Polish mathematician Wacław Sierpiński in 1915.
To visualize its construction, watch this animation:
As we increase the exponent of (¾), the triangles become progressively smaller. When the exponent reaches infinity, we only see the outlines of the triangles. True fractals retain all details no matter how much you zoom in.
Understanding the Full Scope of Fractals
This essay has aimed to provide an intuitive grasp of fractals, starting with fractional decay and moving to the Sierpiński triangle. However, fractals extend beyond geometry and fractions; they can arise in various forms across different mediums, including sound and taste.
Research suggests humans are particularly drawn to fractal patterns that exist within dimensions ranging from 1.3 to 1.5. While we haven’t covered fractal dimensions in detail, think of them as how a fractal behaves differently at various scales.
For now, I’ll conclude with a hint: I often incorporate fractal patterns into my own writing. Take a look at my essay on predicting improbable events, and see if you can spot the pattern in the sub-headings and content.
For further reading, check out: How To Really Understand Recursion and Why LaMDA Is Not Really Sentient.
If you're interested in supporting my work as an author, consider contributing on Patreon.
The second video offers an insightful explanation of fractals and their practical applications.